Materials from Mathematics
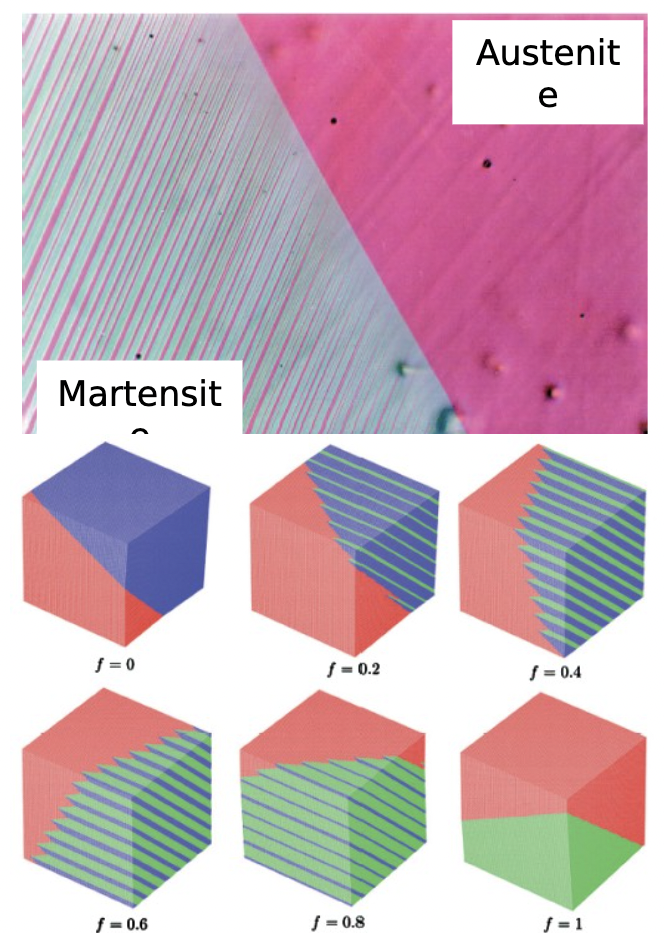
Austenite/martensite interface in Cu69.5Al27Ni3.5. Zero elastic energy austenite/martensite interfaces possible under the co-factor conditions. Red is austenite and blue/green are two variants of martensite. These pictures exhibit large deformations, zero elastic energy, and perfect fitting of the phases under continuous variation of the volume fraction f.
Richard D. James
Largely due to the extreme forms of nonlinearity encountered in the behavior of materials, mathematics and materials science enjoy a healthy interaction. Like some theorems, the discovery of a spectacular new material represents an unmistakable advance, not clouded by shades of meaning. This discussion concerns the mathematical theory that underlies the synthesis of materials that undergo phase transformations, specifically in regard to hysteresis and reversibility. What elements, in what proportion, and with what processing are needed to achieve unprecedented behavior?
Small bubbles of soap froth disappear while big ones grow and grains of a polycrystalline metal coarsen over time. As in the simplest linear elliptic and parabolilc equations, there is a strong tendency to simplify and smooth. However, exactly the opposite happens in a martensitic phase transition. Here, one begins with a uniform crystal of austentite and upon cooling one gets a plethora of fine microstructures of martensite. The mathematical origins of the spontaneous formation of fine structure comprise a fascinating and ongoing chapter of nonlinear analysis.